User:Inconspicuum/Physics (A Level)/Ideal Gases

An animation showing the relationship between pressure and volume when mass and temperature are held constant.

An animation demonstrating the relationship between volume and temperature.

Real-world gases can be modelled as ideal gases. An ideal gas consists of lots of point particles moving at random, colliding with each other elastically. There are four simple laws which apply to an ideal gas which you need to know about:

Boyle's Law

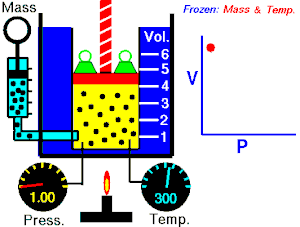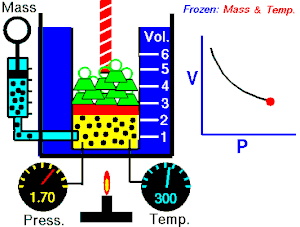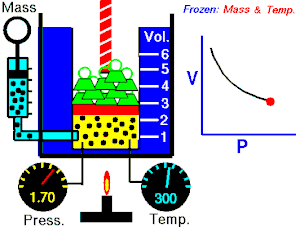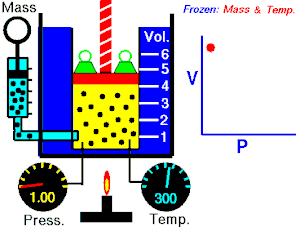
Boyle's Law states that the pressure of an ideal gas is inversely proportional to its volume, assuming that the mass and temperature of the gas remain constant. If I compress an ideal gas into half the space, the pressure on the outsides of the container will double. So:

$p\propto {\frac {1}{V}}$

Charles' Law

Charles' Law states that the volume of an ideal gas is proportional to its temperature:

$V\propto T$

T must be measured in kelvin, where a rise of 1°K is equal to a rise 1°C, and 0°C = 273°K. If we double the temperature of a gas, the particles move around twice as much, and so the volume also doubles.

Amount Law

This law states that the pressure of an ideal gas is proportional to the amount of gas. If we have twice the number of gas particles N, then twice the pressure is exerted on the container they are in:

$p\propto N$

A mole is a number of particles. 1 mole = 6.02 x 10²³ particles. So, the pressure of a gas is also proportional to the number of moles of gas present n:

$p\propto n$

Pressure Law

The pressure law states that the pressure of an ideal gas is proportional to its temperature. A gas at twice the temperature (in °K) exerts twice the pressure on the sides of a container which it is in:

$p\propto T$

These laws can be put together into larger formulae linking p, V, T and N. To do this we require a constant of proportionality, (R) the universal molar gas constant, with an experimental value of 8.31Jmol^-1K^-1