Practical Electronics/Resistors/Series Proof

This page presents a proof that the resistance of a series network, R_tot of n resistors is the sum of the resistance of each resistor in the network:

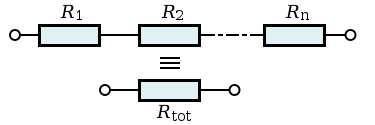
First, consider that a voltage across the whole network must be equal to the sum of the voltages across each resistor (Kirchhoff's Voltage Law).

$v_{tot}=\sum \limits _{k=1}^{n}{v_{k}},$

where v_k is the voltage across the k^th resistor in the network. Now, from Ohm's Law, we can write:

$i_{tot}R_{tot}=\sum \limits _{k=1}^{n}{i_{k}R_{k}},$

where:

• i_tot is the current through the entire network
• R_tot is the resistance of the entire network
• i_k is the current in the k^th resistor in the network
• R_k is the resistance of the k^th resistor in the network

Since from Kirchhoff's Current Law we know that the current in each resistor is the same, we can write

$iR_{tot}=i\sum \limits _{k=1}^{n}{R_{k}},$

where i is the current through the network. Dividing through by i gives us our final answer:

$R_{tot}=\sum \limits _{k=1}^{n}{R_{k}}$

This is a vital result and should be remembered. Notice that the proof for parallel networks is essentially the same, but uses KCL first and KVL second.

Other interesting points are that this result describes behaviour of parallel capacitive networks and series inductive networks.